Vous êtes ici : Accueil > LaTeX > Tikz > Graphe de fonction automatique
Publié : 3 janvier 2018
Format PDF Enregistrer au format PDF

Graphe de fonction automatique

Il suffit de définir les coordonnées du point inférieur gauche et du point supérieur droit pour que le repère cartésien soit automatiquement créé.

L’idée est de "juste" définir les coordonnées des sommets respectivement inférieur gauche et supérieur droit du repère où la fonction doit venir se dessiner.

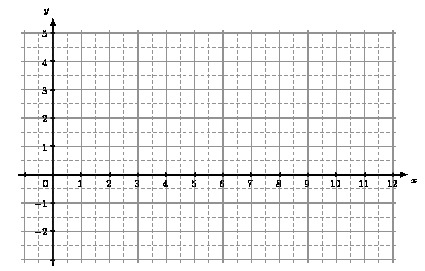
JPEG - 38.7 ko
Repère cartésien avec tikz

Voici le code :

\documentclass[border=5pt]{standalone}
%%%<
\usepackage{verbatim}
%%%>
% \usepackage{pgfplots}
% \pgfplotsset{compat=1.8}
\usepackage{amsmath}
\usepackage{tikz}
\usetikzlibrary{calc}

\begin{document}
%    \begin{center}
          \pgfmathtruncatemacro\xmin{-1}
          \pgfmathtruncatemacro\xmax{12}
          \pgfmathtruncatemacro\ymin{-3}
          \pgfmathtruncatemacro\ymax{5}
          %
                \begin{tikzpicture}[>=latex,scale=1]
                    \draw[very thin,dashed, xstep=0.5, ystep =0.5, color=gray]  ($(\xmin,\ymin) + (-0.1,-0.1)$)  grid ($(\xmax,\ymax) + (0.1,0.1)$) ;
                    \draw[thin,color=gray] ($(\xmin,\ymin) + (-0.1,-0.1)$)  grid ($(\xmax,\ymax) + (0.1,0.1)$) ;
                    %
                    \draw[very thick, ->]  ($(\xmin,0) + (-0.2,0)$)  -- ($(\xmax,0) + (0.5,0)$) node[below right] {$n$};
                    \draw[very thick, ->]  ($(0,\ymin) + (0,-0.2)$)  -- ($(0,\ymax) + (0,0.5)$) node[above left] {$u_n$};
                    %
                    \pgfmathtruncatemacro\xminPlus{\xmin + 1}
                    \pgfmathtruncatemacro\xminPlusDbl{\xminPlus + 1}
                    %
                    \draw[very thick] (\xmin,2pt) -- (\xmin,-2pt);
                    \foreach \x in {\xminPlus,\xminPlusDbl,...,\xmax }{
                        \ifnum\x=0
                          \draw[very thick] (\x,2pt) -- (\x,-2pt)  node[below left, xshift= -0.035cm ] {$\x$} ;
                        \else
                          \draw[very thick] (\x,2pt) -- (\x,-2pt) node[below , xshift= -0.035cm ] {$\x$} ;
                        \fi
                        }
                      %
                        %
%                     \foreach \xpi in {-2,-1,...,2}
%                         \draw[very thick] ({3*\xpi},-2pt) node[below, xshift= -0.007cm ] {${\xpi} \pi$} ;
                        %
                    \pgfmathtruncatemacro\yminPlus{\ymin + 1}
                    \pgfmathtruncatemacro\yminPlusDbl{\yminPlus + 1}
                          \draw[thick] (2pt,\ymin) -- (-2pt,\ymin);
                    \foreach \y in {\yminPlus,\yminPlusDbl,...,\ymax}{
                        %
                        \ifnum\y=0
                          \draw[very thick] (2pt,\y) -- (-2pt,\y);
                        \else
%                          \pgfmathsetmacro\ySurDeuxEtDemi{2*\y/5}
%                           \draw[very thick] (2pt,\y) -- (-2pt,\y) node[ left, yshift= -0.035cm ] {$\pgfmathprintnumber\ySurDeuxEtDemi$} ;
                          \draw[very thick] (2pt,\y) -- (-2pt,\y) node[ left, yshift= -0.035cm ] {$\y$} ;
                        \fi
                        }
                    %
                    %
                      \pgfmathsetmacro\xMinGraphe{\xmin +0.5}
                    %
                      \pgfmathsetmacro\xMaxGraphe{\xmax -0.5}
                    %
%                     \draw[very thick,color=black!40!green!90] plot[samples=200,domain=\xMinGraphe:\xMaxGraphe] (\x,{(3/2)*sin((2*\x  +3.1/3)*(180/3)) +2});
                    %
%                     \draw [pattern color = green, pattern = dots] plot[samples=200,domain=-1.5:2.9]
%                               (\x,{(2*\x +3)^(1/2)}) -- plot[domain=2.9:-1.5] (\x,{2*\x -3}) ;
%                     \draw[thick, dashed, color =orange] (-4,1) -- (4,1) node[above right] {$d \equiv y =1 $};
                \end{tikzpicture}  
%    \end{center}

\end{document}

Documents joints